Sign In Sign-Up

Welcome!

Close

Would you like to make this site your homepage? It's fast and easy...

Yes, Please make this my home page!

No Thanks

  Don't show this to me again.

Close

Hartbeesthoek 94

The New South Africa Datum

Background

The co-ordinate reference system, used in South Africa as the foundation for all surveying, engineering and geo-referenced projects and programmes, is the Cape Datum. This Datum is based on the Clarke 1880 ellipsoid and has its origin point at Buffelsfontein near Port Elizabeth. The Cape Datum is based on the work of HM Astronomers Sir Thomas Maclear, between 1833 and 1870, and Sir David Gill, between 1879 and 1907, whose initial geodetic objectives were to verify the size and shape of the Earth in the Southern Hemisphere and later to provide geodetic control for topographic maps and navigation charts. From these beginnings, this initial network was extended to eventually cover the entire country and now comprises approximately 29 000 highly visible trigonometrical beacons on mountains, high buildings and water towers as well as approximately 20 000 easily accessible town survey marks.

As with other national control survey networks throughout the world, which were established using traditional surveying techniques, flaws and distortions in these networks have become easily detected using modern positioning techniques such as the Global Positioning System (GPS). In addition to these flaws and distortions, most national geodetic networks do not have the centre of their reference ellipsoids co-incident with the centre of the Earth thus making them useful only to their area of application. The upgrading, recomputation and repositioning of the South African co-ordinate system has thus been driven by the advancement of modern positioning technologies and the globalization of these techniques for navigation and surveying.

As from the 1^st January 1999, the official co-ordinate system for South Africa will be based on the World Geodetic System 1984 ellipsoid, commonly known as WGS84, with the ITRF91 (epoch 1994.0) co-ordinates of the Hartebeesthoek Radio Astronomy Telescope used as the origin of the system. This new system will be known as the Hartebeesthoek94 Datum.

At this stage all heights will remain referenced to mean sea level, as determined in Cape Town and verified at tide gauges in Port Elizabeth, East London and Durban.

 

Geoids & Ellipsoids

The earth's physical surface is a tangible one encompassing the mountains, valleys, rivers and surface of the sea. It is highly irregular and not suitable as a computational surface. A more smoothed representation of the earth is the Geoid. There are a number of definitions for this surface; a descriptive one is as follows : ‘that surface that would be assumed by the undisturbed surface of the sea, continued underneath the continents by means of small frictionless channels.'

The Ellipsoid is a smooth mathematical surface that best fits the shape of the geoid and is the next level of approximation of the actual shape of the earth.

Elements of an ellipse a =   Semi Major Axis b =   Semi Minor Axis f =   Flattening = (a-b)/a PP’  =  Axis of revolution  of the earth's ellipsoid

Below is a list of commonly used ellipsoids used in southern Africa and their associated parameters. Ellipsoid                           a                  b                          Unit                          Used

Mod. Clarke 1880	6378249.145	6356514.967	International meters	R.S.A., Botswana,   Zimbabwe
WGS 84	6378137.000	6356752.314	International meters	Globally
Bessel	6377397.155	6356078.963	German Legal meters	Namibia
Clarke 1866	6378206.400	6356584.467	International meters	Mozambique

 

Datums

A National geodetic co-ordinate system is defined by a Geodetic Datum, which consists of two parts:

a) A defined geodetic reference ellipsoid, in terms of the a,b or a,f parameters.

b) A defined orientation, position and scale of the Geodetic system in space.

From this, it can be deduced that a specific ellipsoid can be used to define an infinite amount of datums. This is demonstrated in the figure below.

The Cape Datum

a) The Modified Clarke 1880 is the reference ellipsoid.

b) The initial point for the existing South African Datum is the Buffelsfontein trigonometrical beacon, near Port Elizabeth.

c) The orientation and scale characteristics were defined by periodic astronomic azimuth and base line measurements.

The Hartebeesthoek94 Datum

a) The WGS84 is the reference ellipsoid.

b) The initial point is the Hartebeesthoek Radio Astronomy telescope, near Pretoria.

c) The scale and orientation characteristics were defined within the GPS operating environment and has been confirmed  and has been confirmed to be co-incident with ITRF91 determination.

 

Ellipsoidal Co-Ordinates

The three dimensional (real world) co-ordinates of a point on the earth’s surface can be defined in:

Geographical co-ordinates

Latitude(Ø    : angular displacement north/south of the equator.

Longitude() : angular displacement east/west of the Greenwich meridian.

Height (H)    : either orthometric (height above mean sea level) or ellipsoidal (height above ellipsoid).

Geocentric Cartesian co-ordinates

A three-dimensional Cartesian co-ordinate system (Xg, Yg, Zg) with its origin coinciding with the centre of the reference ellipsoid/Earth, and axes as shown below.

The following are examples of geographical co-ordinates and equivalent Cartesian co-ordinates in South Africa (Cape Datum).

Cape Town
Geographical	34° 12’54" S	18° 30’45"E	Ht   = 0.0m
Cartesian	5006849 Xg	1676482 Yg	-3565925 Zg
Johannesburg
Geographical	26° 20’00" S	28° 12’00" E	Ht 1000.0m
Cartesian	5041892 Xg	2703400 Yg	-2812717 Zg
Durban
Geographical	30° 20’ 00" S	31° 12’ 00" E	Ht = 0.0m
Cartesian	4712938 Xg	2854257 Yg	-3202070 Zg

 

Projected Co-Ordinates

Plane co-ordinates are the simplest type of co-ordinates to use for everyday practical applications. To achieve this simplicity, the ellipsoidal latitude and longitude co-ordinates, or 3-D geocentric co-ordinates, must therefore be projected onto a plane surface. It is not possible to do this without some distortion. This can be demonstrated by cutting a tennis ball in half and attempting to flatten it.

Projections which have the properties of preserving angles and shapes are called Conformal or Orthomorphic projections. In South Africa the Gauss Conform Projection (an adaptation of the Transverse Mercator projection) is used for the computation of the plane YLo, XLo co-ordinates, commonly known as the "Lo. co-ordinate system".

Here the equator will project as a straight line, at right angles to the central meridian (Lo.), but all other meridians and parallels will project as curved lines. The equator and the Lo. are the origins of the Y_Lo and X_Lo axes of our plane rectangular co-ordinate system. The figure, above, shows the relationship between plane (Lo.) co-ordinates and geographical co-ordinates.

In the South African plane co-ordinate system only the area within one degree of longitude on either side of the central meridian is projected. The width of each segment, often referred to as a belt, is thus two degrees of longitude and is referred to the central meridian of that belt.

 

Datum Relationships

Geocentric Cartesian co-ordinates in South Africa

For comparative purposes the simplest form of transformation, being a geocentric Cartesian translation between the two Datums’ ellipsoid geocentres, will be used

Figure - The ellipsoid relationships

The translations were determined by computing the Hartebeesthoek94 Datum and the Cape Datum co-ordinates of a number of accurately determined trigonometrical beacons.

Note:

These transformation parameters will yield co-ordinates in the other datum with residuals that should not exceed 15 metres.

Geographical co-ordinates in South Africa

The Hartebeesthoek94 Datum(WGS84) latitude is always numerically greater than its Cape Datum(modified Clarke 1880) counterpart at a point of interest. The magnitude of this difference ranges from approximately 9" at the equator to approximately 0" at latitude 37° South.

The Hartebeesthoek94 Datum(WGS84) longitude is always numerically less than its Cape Datum(modified Clarke 1880) counterpart at a point of interest. The magnitude of this difference ranges from approximately 2" at 15° east of Greenwich to 0" at approximately 39° east of Greenwich.

Gauss conform co-ordinates (Lo system) in South Africa

The Hartebeesthoek94 Datum(WGS84) X_Lo co- ordinate is between 290 and 300 metres greater than its Cape Datum(modified Clarke 1880) counterpart at a point of interest. This difference is directly related to the displacement in the equatorial planes of two ellipsoids.

The Hartebeesthoek94 Datum(WGS84) Y_Lo co-ordinate will always be algebraically greater than its Cape Datum(modified Clarke 1880) counterpart, at a point of interest. The magnitude of this difference ranges from approximately 70 metres at 15° east of Greenwich to 0 metres at approximately 39° east.

The relationship between the longitude differences and Gauss conform Y_Lo co-ordinate differences are opposite in sign. The reason for this is that longitude, by convention, increases eastward and the projection Y_Lo co-ordinate, by definition, increases westwards. Effectively the relationship is the same.

 

Who will be Affected

a)	Hikers and Navigators
	This group of users are least effected since the accuracy they require generally exceeds 50m. They should at least be aware of the how the two Datums’ relate to each other.
b)	GIS practitioners and other users of Geospatial Information Databases
	In general,these users require a level of accuracy not better than 10cm. The impact on GIS databases depends on :
	1. The accuracy of the co-ordinates in the database at present.
	2. The purpose of the database.
	3. The extent of the database (e.g. local authority, provincial, national).
c)	Surveyors, Engineers, Architects etc.
	In general,these users require data to the centimetre level of accuracy .This category of users will be most affected and must follow a meticulous approach.

 

Transformation Strategy

Although the advantages of adopting a new horizontal datum are evident, many users have huge geographically referenced datasets based on the Cape Datum and need to transform these to the new datum. To do this, one needs to establish the relationship between the two datums as this is not absolutely defined.

Various methods of transformation are available in order to transform current data sets, referenced with respect to the Cape Datum, to the new Hartebeesthoek94 Datum.

Because of computational limitations, there are many distortions in the Cape Datum co-ordinates which have been removed in the computation of Hartebeesthoek94 Datum co-ordinates. For this reason the higher the transformation accuracy required, the more localised the area must be for which transformation parameters are calculated. For high end users, such as Land Surveyors and Engineers, transformation accuracy is of paramount importance. Low accuracy GIS applications may however not require this accuracy and parameters covering larger areas may be acceptable.

Transformation parameters

In computing transformation parameters one would require co-ordinates of control points in both datums. The 29 000 trigonometrical beacons and 22 000 town survey marks will serve this purpose adequately.

At least two (for 2-D) or three (for 3-D) points, known in both the old and the new Datum, must be used to determine the transformation parameters and must be well distributed through the area to be transformed.

The Chief Directorate: Surveys and Mapping has evaluated two-dimensional Helmert (4 parameter) transformation parameters, derived from the horizontal control survey network data within the two datums, over various sized areas. The averaged residuals obtained are as follows:

The most common transformation models are Helmert ( for two dimensional transformations) and Bursa Wolfe (for three dimensional transformations).

Two dimensional ( Helmert transformation model)
system 2	system 1
Y2      =         TY + b.X1 + a.Y1
X2       =         TX + a.X1 - b.Y1
The transformation parameters to solve for are therefore:
TX, TY : The translations in each axis.
a, b   : Helmert transformation variables.

An Example

Transforming a co-ordinate (on Lo19º) from Cape Datum to the Hartebeesthoek94 Datum using predetermined parameters.

Three Dimensional (Bursa Wolfe transformation model)

system 2                                 system 1

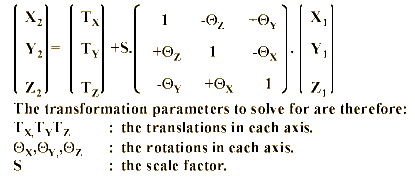
An Example

Transforming a co-ordinate (on Lo27º) from the Cape Datum to the Hartebeesthoek94 Datum using predetermined parameters.

 

Conclusion

The Cape Datum has served its purpose well over the last century. With the advent of artificial satellite surveying techniques this Datum has proved to be inadequate for our future needs. The introduction of the Hartebeesthoek94 Datum will ensure that South Africa remains at the forefront of modern geodetic networks and provides us with a Datum that is internationally related and relevant to our user needs.

 

What will be Available to Users?

• A helpdesk at the office of the Chief Directorate: Surveys and Mapping.
• Co-ordinate lists of all trigonometrical beacons and town survey marks on the Hartebeesthoek94 datum (WGS84 ellipsoid).
• Co-ordinates on the Cape datum (mod. Clarke 1880 ellipsoid ) will be made available for reference purposes.
• Equations/algorithms of required conversions and transformation to assist users wishing to carry their own conversions/transformations.
• Software to transform ASCII co-ordinate files from the Cape Datum to the Hartebeesthoek94 Datum, referred to as "The 1999 Datum Transformation Software", aimed at supporting GIS users.
• Revised and updated triangulation and town survey plans.
• Please note that mean sea level (MSL) will remain the reference surface for all heighting purposes.

 

Further information, please contact :

R. Wonnacott, WGS84 Co-ordinator
Telephone:	+27 (0)21 685 4070
Fax:	+27 (0)21 689 1351
E-mail:	dmapping@sli.wcape.gov.za
Address:	Private Bax X10, Mowbray, 7705 South Africa